1. A microwave oven heats by radiating food with microwave
energy, which is absorbed by
the food and converted to heat. Suppose such an oven's radiation
wavelength is 10.0 cm. A
container with 200 g of water was placed in the oven, and the
temperature of the liquid water
rose from 20.0oC to 90.0oC. How many photons
of this microwave
radiation were required? Assume that all of the energy of the
radiation was used to raise the
temperature of the water.
Ephoton = hc/(lambda)
Ephoton = [6.62 x 10-34 J s x 3.00 x
108 m/s x
102 cm/m]/10.0 cm
= 1.99 x 10-24 J
This is the energy supplied by one photon, expressed as
J/photon.
Energy required to warm the water is:
E = 200 g x 4.184 J/(g deg) x 70.0 deg = 5.86 x 104
J
Thus, # photons required = [5.86 x 104 J]/[1.99 x
10-24 J/photon]
number of photons required = 2.94 x 1028
2. Using bond energy data, determing whether the reaction
HCN(g) + 2H2(g) = CH3NH2(g)
is endothermic or exothermic. Be sure to draw Lewis structures
for all reactants and
products. Note that CH3NH2 has H of
NH3 replaced by
a CH3 group.
When one draws appropriate Lewis structures, it can be seen that
the bonds to be broken (in
the reactants) are hydrogen-carbon single bonds, carbon-nitrogen
triple bonds, and
hydrogen-hydrogen single bonds. As well, the bonds to be
formed (in the products) are
carbon-hydrogen single bonds, carbon-nitrogen single bonds, and
nitrogen-hydrogen single
bonds.
Delta H = Sum of bond enthalpies of reactants - Sum of bond
enthalpies of products.
Following Kotz and Treichel, we designate bond enthalpies by D.
Delta H = DH-C + DC-N (triple bond) + 2
DH-H -
DC-N - 3 DH-C - 2 DN-H.
Delta H = [414 + 891 + 2(436) - 293 - 3(414) - 2(389)]kJ
Delta H = -136 kJ (exothermic)
3.a) In each of the following sets, which atom or ion has the
smallest radius?
i) O+, O, O-
Cation is smaller than atom; anion is larger than atom.
O+
is smallest.
ii) S, Cl, Se
Size decreases across a period, and increases down a group. Cl
is smallest.
iii) Li, N, F
Size decreases across a period. F is smallest.
b) In each of the following sets, which atom or ion has the
smallest ionization energy?
i) Ne, Cl, Ar
I.E. decreases as go down a group, and increases as go across
a period. Cl has smallest I.E.
ii) Rb, Cs, Ba
I.E. decreases as go down a group, and increases as go across
a period. Cs has smallest I.E.
iii) O2-, O, O-
All three species have the same nucleus, but have differing number
of electrons. O2- has smallest I.E.
c) Using data from Chapter 8 of Kotz and Treichel, determine,
in kJ/mol, the ionization energy of Cl- and the electron
affinity of Cl+.
The ionization energy of Cl- refers to Cl-(g)
--> Cl(g) + e-
This process is the reverse of the E.A. for Cl(g).
I.E. here = +349.0 kJ/mol
The electron affinity for Cl+ refers to Cl+
+ e- --> Cl(g)
This process is the reverse of the I.E. for Cl(g)(see Fig
8.13).
E.A. here = -1251 kJ/mol
4. For the following molecules and ions (note that both
HPO4- and
HSO3- have H bonded to O):
F2O, HPO42-,
SeF4, IBr3, HSO3-,
BrO3-
a) draw Lewis structures (including resonance forms where
appropriate);
b) categorize each species according to the
AXnEm
nomenclature;
c) name the shape of the species;
d) predict whether those species which are neutral molecules will
have a dipole moment;
e) for all the species that contain oxygen atoms, calculate the
bond order of the central atom to oxygen bonds.
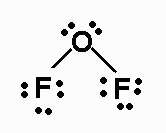
F2O
F2O has 20 valence electrons. Its Lewis structure is

The molecule is AX2E2, and has a bent
geometry.
It has a dipole moment. The two O-F bonds have B.O. = 1.
HPO42-
HPO42- has 32 valence electrons. Its Lewis
structure is

The ion is AX4 around P, and has a tetrahedral geometry.
B.O. = 1.0 for P-O of P-O-H, and B.O. = 4/3 for P-O with no H.
SeF4
SeF4 has 34 valence electrons. Its Lewis structure
is
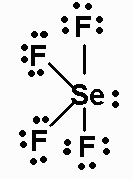
The molecule is AX4E, and has a seesaw geometry. It
has a dipole moment.
IBr3
IBr3 has 28 valence electrons. Its Lewis structure
is
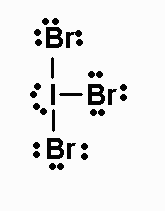
The molecule is AX3E2, and has a T-shaped
geometry. It has a dipole moment.
HSO3- (H is bonded to O);
HSO3- has 26 valence electrons. Its Lewis
structure is
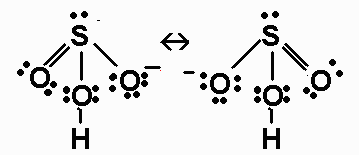
The molecule is AX3E around S, and has a triangular
pyramidal geometry around S. The S-O B.O. for
the S-O-H bond is 1.0; the other S-O B.O.s are 1.5.
BrO3-
BrO3- has 26 valence electrons. Its Lewis
structure is
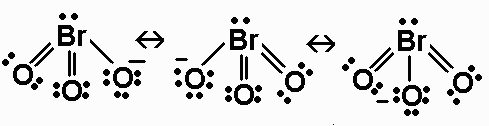
The ion is AX3E, and has a triangular pyramidal
geometry.
The Br-O B.O. is 5/3.
5. Draw resonance structures and predict bond orders and bond
angles for the following:
a) NO2Cl (N is the central atom);
NO2Cl has 24 valence electrons. Its Lewis structure
is

N-Cl has a B.O. of 1.0; N-O has a B.O of 1.5; all bond angles
are 120o.
b) SO32-.
SO32- has 26 valence electrons. Its Lewis
structure is

S-O B.O. = 4/3. All bond angles are less than 109.5o.
6. Place the species below in the order of increasing bond
length for the N-O bond;
H2NOH (N is the central atom, and one H is bonded to
O), NNO,NO+, NO2-,
NO3-.
The Lewis structures are:
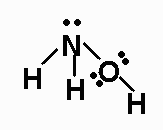
The N-O B.O. for H2NOH is 1.0.
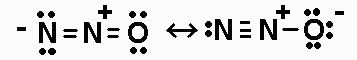
The N-O B.O.for NNO is 1.5.
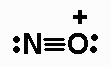
The N-O B.O. for NO+ is 3.0.
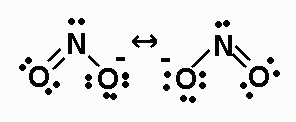
The N-O B.O. for NO2- is 1.5.
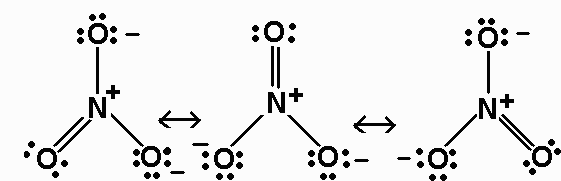
The N-O B.O.for NO3- is 4/3.
The order of increasing bond lengths is: NO+ <
NO2- = NNO < NO3- <
H2NOH.
7. 1.00 g PCl5 is introduced into a 250 mL flask, the
flask is heated to
250oC, and the dissociation of PCl5 is
allowed to reach equilibrium
according to PCl5(g) = PCl3(g) +
Cl2(g). The quantity of
Cl2(g) present at equilibrium is found to be 0.250 g.
What is the value of the
equilibrium constant Kc for this reaction at
250oC?
1.00 g/(208.2 g/mol) = 4.80 x 10-3 moles
PCl5
Set up an array of moles, and then convert to concentrations, to
find Kc.
| MOLES | PCl5 | PCl3 | Cl2 |
| Initial | 4.80 x 10-3 | 0 | 0 |
| Change | -x | x | x |
| Equilibrium | 4.80 x 10-3 -x | x | x |
We are given that x = 0.250 g/(70.90 g/mol) = 3.53 x
10-3 moles.
Kc = {(x/0.250)2}/{(4.80 x 10-3
-x)/0.250}
Kc = 3.92 x 10-2 mol/L
8. For the equilibrium CaCO3(s) = CaO(s) +
CO2(g),
Kp
= 1.16 atm at 800 oC. If 20.0 g of CaCO3 is
put into a 10.0 L
container and heated to 800 oC, what percent of the
CaCO3 would
remain undissociated at equilibrium?
Kp = PCO2
One can calulate the equilibrium moles of CO2 from PV =
nRT:
n = PV/RT = [(1.16 atm)(10.0 L)]/[(0.0821 L atm mol-1
K-1)(1073 K)]
= 0.132 mol
moles CaCO3 initially present = 20.0 g/(100.1 g/mol) =
0.200 mol
% unreacted = (moles unreacted)/(moles originally present) x
100%
% unreacted = (0.200-0.132)/(0.200) x 100 % = 34.0 %